Donald in Mathmagic Land
- timothyharleywright
- Mar 6, 2020
- 2 min read
When I was in grade school, we watched this movie half a dozen times. As an adult, I was determined to incorporate it on my website.
Then I found out it’s available online.
I was particularly fascinated by the segment about the “Golden Section” and the five-pointed star. I will say more about this below.
Donald in Mathmagic Land
The Golden Section, a/k/a “Phi”
This presentation poses challenges for me, given the jumping-through-hoops involved in displaying algebra, fractions and geometric figures online. It includes errors I won’t fix.
<———————————— a + b ——————————> ooooooooooooooooooooooooooooooooooxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxx <————— a ————><—————— b ———————>
The Golden Section is the division of a line segment at a point such that the short part is to the long part, as the long part is to the whole. In other words,
a = b b a + b
The ancients believed that figures based on this ratio were the most harmonious of all, in part because the ratio incorporates both addition and multiplication, which respectively express the cosmic principles of masculinity and femininity. (I may have that backwards.) Thus this ratio embodies cosmic sexual bliss.
What is its numerical value?
Clearing the denominators,
a² + ab = b²
Substituting 1 for a:
1 + b = b²
This deserves to be a curiosity in itself. Put in standard form:
0 = b² − b − 1
Applying the quadratic formula yields this:
b = (½)√5̅ ± ½
Or, one could say:
b = (½)(5)(1/2) ± ½
So there are two values for b. The Golden Ratio being generally known as Phi (the name of the Greek letter Φ, pronounced “fee”), I will call the smaller value phi and the larger value PHI.
√5̅ = 2.360678 (½)√5̅ = 1.1180339 (½)√5̅ + ½ = 1.6180339 = PHI (½)√5̅ − ½ = 0.6180339 = phi
The relation of these numbers to the five-pointed star, begins with the trigonometry of an angle of 18 degrees. Sin (18) = 0.309017, half of phi.
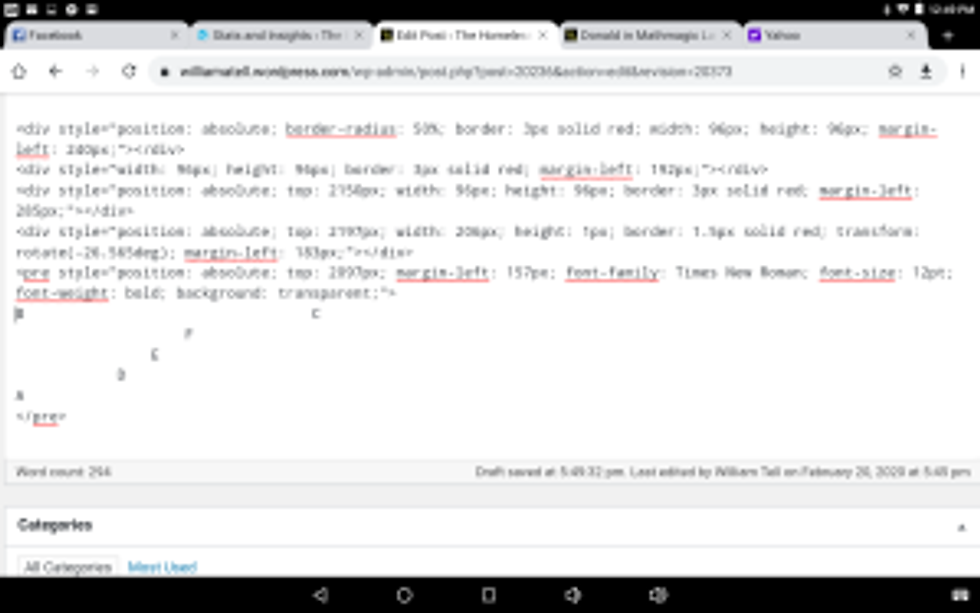
The simplest way I know of to construct a demonstration of the Golden Section, is to reproduce this figure:
1. Draw two squares side by side. 2. Draw a diagonal from the lower left corner to the upper right. 3. Draw a circle around the centerline.
Length AB being 1, and length BC being 2, length AC must be √5̅.
Length AE, then, is (½)√5̅.
The radius of the circle is 1/2.
Thus length AD = (½)√5̅ − 1/2 = phi, and length AF = (½)√5̅ + 1/2 = PHI.
The DaVinci Code
For those who may have interest, here is the HTML for the above figure:

Comentarios